Index to this page

Antibody Affinity

The strength with which an antibody molecule binds an epitope (= antigenic determinant) is called its affinity.

All the interactions between the two contribute to it. These are noncovalent interactions:

ionic interactions like attraction of opposite charges on amino acids;
hydrogen bonds;
hydrophobic interactions.

Affinity can be quantified by determining an association constant, K.

Equilibrium dialysis is a technique for doing this.

The procedure requires that the epitope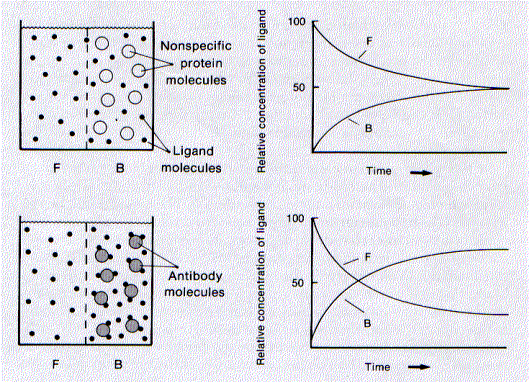

be present on a molecule — the ligand — small enough to pass through the pores of a membrane (like a sheet of cellophane);
be labeled in some way.

In the example I shall give, the ligand

is a soluble hexasaccharide derived from the Type III polysaccharide capsule — which consists of alternating units of glucose and glucuronic acid — that coats one common strain of pneumococci;
is labeled with the radioactive isotope tritium (³H).

This figure shows how equilibrium dialysis performed.

TOP: where there is no interaction between the ligand and the protein in the "Back" (B) chamber.
- The radioactive hexasaccharide — dissolved in a suitable buffer — is placed in the front chamber ("F").
- Serum from a nonimmune animal (e.g., a rabbit) is placed in the back chamber ("B").
- Periodically, samples are removed from each side and their radioactivity measured.
- At first, all the radioactivity is in F, but as diffusion proceeds, the radioactivity — and thus the concentration of the ligand — declines in F as it rises in B.
- After a few hours, the concentration of ligand in the two chambers becomes equal and remains so.
BOTTOM: where the ligand is bound by antibodies in the B chamber.
- Same as before, except that the Back (B) chamber is filled with a buffer solution containing antibodies against the Type III polysaccharide.
- This time, the concentration of ligand in B rises to an equilibrium value higher than its concentration in F.
- The difference between the two represents the ligand that has bound to antibody molecules and, while bound, is unable to diffuse back into chamber F.

For a given concentration of antibody molecules, the greater the fraction of ligand bound by them, the greater their affinity for the ligand. This value can be expressed as an association constant, K. At equilibrium

K =     [Ab–Lg]
          ‾‾‾‾‾‾‾‾‾
           [Ab] [Lg]

where the terms in brackets represent the concentration of

antibody-ligand complexes [Ab–Lg],
unbound antibody [Ab], and
unbound ("free") ligand [Lg].

(Note that this is the reciprocal of the classical dissociation constant.)

A more convenient form of this equation is the Scatchard equation

K =           r
           ‾‾‾‾‾‾‾
           (n − r)(c)

where

r = the ratio of the concentration of bound ligand to the concentration of antibody molecules placed in the system,
n = the number of ligand binding sites on the antibody molecule (i.e., its valence), and
c = the concentration of unbound ("free") ligand.

This table gives the setup and results of equilibrium dialysis performed on a rabbit anti-pneumococcal antiserum diluted in buffered saline to a final concentration of 1.82 µM (1.82 x 10^-6 M).

A ³H-labeled hexasaccharide derived from the capsule of the Type III pneumococci used to immunize the rabbit was added to a series of chambers in concentrations ranging from 0.2 to 6.4 µM.
When equilibrium was reached, the radioactivity was measured — in counts per minute (cpm) — for samples taken from the F and B chambers of each dialysis apparatus.
The radioactivity in B represents
- the concentration of unbound ligand — at equilibrium — equal to that in the other chamber (F) PLUS
- a concentration of ligand bound to antibody molecules in B.

A	B	C	D	E	F⁽¹⁾	G⁽²⁾	H⁽³⁾	I	J⁽⁴⁾
Ligand added (x 10^-6M)	"F"ront cpm	"B"ack cpm	(B+C) Total cpm	(C-B) Bound cpm	Bound ligand (x 10^-6M)	Free ligand (c) (x 10^-6M)	r	r/c (x 10⁶)	K (x 10⁶ M^-1)
0.2	182	1044	1226	862	0.14	0.03	0.08	2.6	1.4
0.4	367	2021	2388	1654	0.28	0.06	0.15	2.5	1.4
0.8	739	3923	4662	3184	0.55	0.13	0.30	2.4	1.4
1.6	1616	7394	9010	5778	1.0	0.29	0.57	2.0	1.4
3.2	3952	14347	18299	10395	1.8	0.69	1.0	1.5	1.4
6.4	11341	27028	38369	15687	2.6	1.9	1.4	0.8	1.4
"B"ack chamber contained anti-type-3 antibodies at a concentration of 1.8 x 10^-6M
(1) (E/D) x A		(2) (B/D) x A		(3) F/1.8 x 10^-6 M		(4) r/[(2−r) x c]

To determine the c, we multiply the concentration of ligand that was initially added by the ratio of radioactivity (in cpm) in chamber F to the total radioactivity.
To determine the concentration of bound ligand, we
- subtract the radioactivity in F from that in B.
- The ratio of bound cpm to total cpm is then multiplied by the concentration of ligand initially added to give the concentration of bound ligand.
To determine r, we simply divide this value by the concentration of antibody molecules (in this case 1.8 µM).

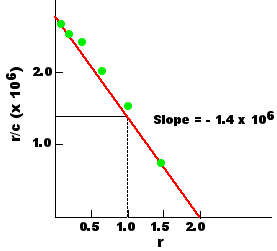
A useful way of presenting the resulting data is to plot the ratio r/c as function of r. If K is truly a constant, such a plot — called a Scatchard plot — should produce a straight line, the slope of which is − K. This Scatchard plot shows that the antibodies had an affinity for the ligand of 1.4 x 10⁶ liters/mole (M⁻¹).

A second useful piece of information revealed by the plot is that the maximum value for r (the x intercept) is 2. At infinitely high concentrations of ligand, each antibody molecule can bind a maximum of 2 ligand molecules; that is, the valence of these antibodies is 2. (They were IgG antibodies.)

Antisera

The fact that all the antibody molecules in this experiment have the same affinity is unusual.

The immune response to most antigens produces an antiserum — a heterogeneous mixture of antibodies. Even though they all are capable of binding the antigen, they represent a pooled mixture of antibodies derived from many different clones, each with a unique B-cell receptor (BCR) — with a unique affinity — for the antigen.

In this case, the rabbits had been immunized in such a way that a single clone of antibody-secreting plasma cells had come to dominate the response. Ordinarily, only monoclonal antibodies made in the laboratory would give a Scatchard plot like this one.

This next table shows the results of equilibrium dialysis performed on a more typical antiserum. K is no longer a constant, but ranges from a value of

0.8 x 10⁶ M⁻¹ in the chambers to which 5 µM ligand was added to
0.4 x 10⁶ M⁻¹ in the chambers to which 50 µM ligand was added.

A	B	C	D	E	F⁽¹⁾	G⁽²⁾	H⁽³⁾	I	J⁽⁴⁾
Ligand added (x 10^-6M)	"F"ront cpm	"B"ack cpm	(B+C) Total cpm	(C-B) Bound cpm	Bound ligand (x 10^-6M)	Free ligand (c) (x 10^-6M)	r	r/c (x 10⁶)	K (x 10⁶ M^-1)
5	400	4144	4544	3744	4.1	0.44	0.52	1.2	0.8
10	1262	8006	9268	6744	7.3	1.4	0.92	0.68	0.63
15	2702	11348	14052	8644	9.2	2.9	1.2	0.40	0.49
25	6648	18159	24807	11511	12.	6.7	1.5	0.22	0.41
40	13693	27380	41073	13687	13.	13.	1.7	0.13	0.40
50	19286	34091	53377	14805	14.	18.	1.8	0.10	0.40
"B"ack chamber contained anti-type-3 antibodies at a concentration of 7.9 x 10^-6M
(1) (E/D) x A		(2) (B/D) x A		(3) F/7.9 x 10^-6 M		(4) r/[(2−r) x c]

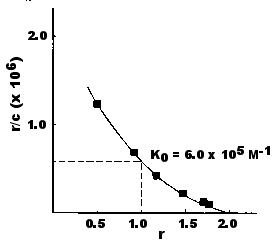
The heterogeneity of binding in this experiment is also revealed by this Scatchard plot of these data. Now the slope of the line is continuously changing as the concentration of ligand in the chambers increases.

Scatchard plots like this are the norm, not the exception, for antisera.

Despite the heterogeneity of affinities in most antisera, it is convenient to characterize such populations by defining an average affinity (K₀). K₀ is the value of K when the ligand concentration is such that one-half the antigen-binding sites are filled. For bivalent antibodies (like the IgG antibodies tested here), this would occur when r = 1. Thus,

K₀ =         1
           ‾‾‾‾‾‾‾
           (2 − 1) c

or 1/c.

Thus, the average affinity is the reciprocal of the concentration of free ligand (c) when r = 1. This value can be read directly from a Scatchard plot because when r = 1, r/c = 1/c = K₀. For the antiserum tested above, K₀ = 6.0 x 10⁵ M⁻¹.

Affinity Maturation

The data presented in the table and in the Scatchard plot above can be transformed according to the Sips equation

log [r/(2−r)] = a log K + a log c

where r, K and c retain their earlier definitions.

Plotting log [r/(2−r)] as a function of log c yields a straight line. (The coefficient a is slope of the line). Once again, the value for K₀ can be read directly from the plot: when r =1, log [r/(2−r)] = 0. Therefore K₀ is the reciprocal of that value of c where the log [r/(2−r)] = 0.

The average association constant (K₀) of an antiserum tends to rise with time following immunization. This phenomenon is called affinity maturation. Presumably, as new B cells expressing new BCRs arise, they are selected for if their BCR has a greater affinity for the antigen. As they develop into a clone of plasma cells, their secreted BCRs — their antibodies — raise the K₀ of the antiserum.

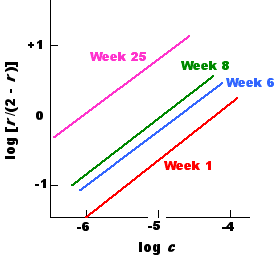
This graph shows the Sips plots of the serum samples taken at four different times from a single rabbit immunized with the Type III pneumococcal polysaccharide. The average association constant, K₀, rose from 1.6 x 10⁴ M⁻¹ at week 1 to 6 x 10⁵ M⁻¹ at week 25.

Affinity maturation reflects an adaptive response to antigen exposure. As time goes by, the antibodies produced are able to bind antigen more tightly and to deal more efficiently with the antigen.

However, this is not the same as saying — as is so often seen — that the antiserum has become more specific. Often an antibody with a very high affinity for one epitope will have a substantial affinity for a related epitope as well. Such an antibody — while highly efficient — would actually have a low specificity.

Antibody Specificity

The specificity of an antibody is its ability to discriminate between two different epitopes.

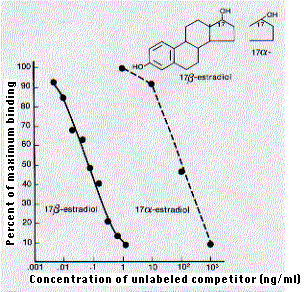
The specificity of antibodies can be so precise that they are able to discriminate between enantiomers of the same molecule. The major estrogen found in women of reproductive age is 17β-estradiol.

But as this graph [taken, with permission, from W. M. Hunter, "Radioimmunoassay" in Handbook of Experimental Immunology, 3rd ed., Vol. 1, Blackwell, 1978.] shows, the shift of the hydroxyl group on carbon 17 from the beta position (extending above the plane of the molecule) to the alpha position (extending below) lowers by 1000-fold the affinity of the molecule for antibodies raised against 17β-estradiol. (The binding was measured by radioimmunoassay.)

Welcome&Next Search

20 December 2010